Graph structure
In July 2016, Cosmin Ionita and Pat Quillen of MathWorks used MATLAB to analyze the Math Genealogy Project graph. At the time, the genealogy graph contained 200,037 vertices. There were 7639 (3.8%) isolated vertices and 1962 components of size two (advisor-advisee pairs where we have no information about the advisor). The largest component of the genealogy graph contained 180,094 vertices, accounting for 90% of all vertices in the graph. The main component has 7323 root vertices (individuals with no advisor) and 137,155 leaves (mathematicians with no students), accounting for 76.2% of the vertices in this component. The next largest component sizes were 81, 50, 47, 34, 34, 33, 31, 31, and 30.
For historical comparisonn, we also have data from June 2010, when Professor David Joyner of the United States Naval Academy asked for data from our database to analyze it as a graph. At the time, the genealogy graph had 142,688 vertices. Of these, 7,190 were isolated vertices (5% of the total). The largest component had 121,424 vertices (85% of the total number). The next largest component had 128 vertices. The next largest component sizes were 79, 61, 45, and 42. The most frequent size of a nontrivial component was 2; there were 1937 components of size 2. The component with 121,424 vertices had 4,639 root verticies, i.e., mathematicians for whom the advisor is currently unknown.
Top 25 Advisors
Most Descendants
| Name | Descendants | Year of Degree |
|---|---|---|
| Abu Mansur al-Hasan ibn Nuh al-Qumri | 242749 | |
| Abu Sahl 'Isa ibn Yahya al-Masihi | 242749 | |
| Abu Abdallah Al-Husayn ibn Ibrahim al-Natili | 242749 | |
| Abu ʿAli al-Husayn (Avicenna) ibn Sina | 242748 | |
| Bahmanyār ibn al-Marzubān | 242747 | |
| Ghiyāth al-Dīn Abū al-Fatḥ ʿUmar ibn Ibrāhīm al-Khayyām al-Nīsābūrī | 242746 | 1068 |
| Saraf al-Dīn Muhammad al-Masʿūdī al-Marwazī | 242745 | |
| Sharaf al-Dīn al-Ṭūsī | 242743 | |
| Fakhr al-Dīn Muhammad al-Rēzī | 242743 | |
| Kamāl al-Dīn Ibn Yūnus | 242742 | |
| Qutb al-Dīn Ibrāhīm al-Mīṣrī | 242742 | 1222 |
| Athīr al-Dīn al-Mufaḍḍal al-Abharī | 242741 | 1264 |
| Nasir al-Dīn al-Ṭūsī | 242740 | |
| Shams al‐Dīn al‐Bukhārī | 242737 | |
| Gregory Chioniadis | 242736 | 1296 |
| Manuel Bryennios | 242735 | 1300 |
| Theodore Metochites | 242734 | 1315 |
| Gregory Palamas | 242731 | 1316 |
| Nilos Kabasilas | 242730 | 1363 |
| Demetrios Kydones | 242729 | |
| Elissaeus Judaeus | 242704 | |
| Georgios Plethon Gemistos | 242703 | 1380, 1393 |
| Basilios Bessarion | 242700 | 1436 |
| Manuel Chrysoloras | 242691 | |
| Giovanni Conversini | 242691 | 1363 |
Nonplanarity
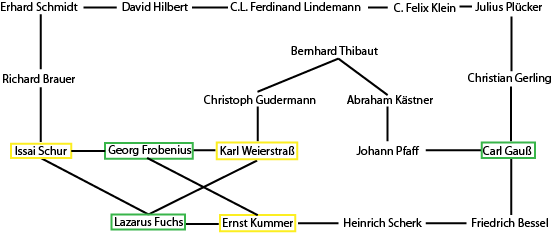
The Mathematics Genealogy Project graph is nonplanar. Thanks to Professor Ezra Brown of Virginia Tech for assisting in finding the subdivision of K3,3 depicted below. The green vertices form one color class and the yellow ones form the other. Interestingly, Gauß is the only vertex that needs to be connected by paths with more than one edge.
Frequency Counts
The table below indicates the values of number of students for mathematicians in our database along with the number of mathematicians having that many students.
| Number of Students | Frequency |
|---|---|
| 0 | 252702 |
| 1 | 34310 |
| 2 | 12535 |
| 3 | 7195 |
| 4 | 4909 |
| 5 | 3728 |
| 6 | 2869 |
| 7 | 2310 |
| 8 | 1910 |
| 9 | 1562 |
| 10 | 1311 |
| 11 | 1102 |
| 12 | 965 |
| 13 | 844 |
| 14 | 675 |
| 15 | 619 |
| 16 | 558 |
| 17 | 478 |
| 18 | 378 |
| 19 | 347 |
| 20 | 332 |
| 21 | 257 |
| 22 | 254 |
| 23 | 242 |
| 25 | 198 |
| 24 | 193 |
| 26 | 171 |
| 28 | 142 |
| 27 | 139 |
| 30 | 110 |
| 29 | 107 |
| 31 | 84 |
| 32 | 73 |
| 34 | 70 |
| 33 | 69 |
| 36 | 68 |
| 35 | 59 |
| 37 | 46 |
| 38 | 41 |
| 39 | 41 |
| 42 | 36 |
| 43 | 35 |
| 41 | 31 |
| 40 | 27 |
| 45 | 27 |
| 46 | 27 |
| 44 | 23 |
| 52 | 21 |
| 50 | 19 |
| 47 | 18 |
| 49 | 18 |
| 48 | 15 |
| 54 | 15 |
| 53 | 14 |
| 55 | 13 |
| 51 | 12 |
| 56 | 12 |
| 57 | 12 |
| 58 | 10 |
| 64 | 10 |
| 60 | 9 |
| 59 | 8 |
| 61 | 8 |
| 63 | 7 |
| 65 | 7 |
| 72 | 7 |
| 68 | 6 |
| 69 | 6 |
| 70 | 6 |
| 74 | 6 |
| 82 | 6 |
| 66 | 5 |
| 73 | 5 |
| 78 | 5 |
| 62 | 4 |
| 75 | 3 |
| 80 | 3 |
| 85 | 3 |
| 67 | 2 |
| 71 | 2 |
| 77 | 2 |
| 79 | 2 |
| 90 | 2 |
| 93 | 2 |
| 76 | 1 |
| 81 | 1 |
| 84 | 1 |
| 88 | 1 |
| 94 | 1 |
| 95 | 1 |
| 97 | 1 |
| 99 | 1 |
| 100 | 1 |
| 101 | 1 |
| 102 | 1 |
| 105 | 1 |
| 107 | 1 |
| 111 | 1 |
| 127 | 1 |
| 130 | 1 |
| 133 | 1 |
| 146 | 1 |
| 181 | 1 |